Mutual arrangement of planes, angle between planes.

Condition of parallelism of two planes:

Let $P_1:A_{1}x+B_{1}y+C_{1}z+D_1=0,$ ${\bar{N}}_1=(A_1,B_1,C_1);$

$P_2:A_{2}x+B_{2}y+C_{2}z+D_2=0,$ ${\bar{N}}_2=(A_2,B_2,C_2).$

Planes $P_1$ and $P_2$ are parallel if and only if ${\bar{N}}_1\parallel {\bar{N}}_2\iff$ $\frac{A_1}{A_2}=\frac{B_1}{B_2}=\frac{C_1}{C_2}.$

Condition for perpendicularity of two planes:

Let $P_1:A_{1}x+B_{1}y+C_{1}z+D_1=0,$ ${\bar{N}}_1=(A_1,B_1,C_1);$

$P_2:A_{2}x+B_{2}y+C_{2}z+D_2=0,$ ${\bar{N}}_2=(A_2,B_2,C_2).$

$P_1\perp P_2\iff$ ${\bar{N}}_1\perp {\bar{N}}_2\iff$ $A_1\cdot A_2+B_1\cdot B_2+C_1\cdot C_2=0.$

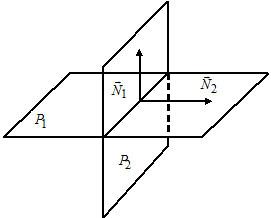
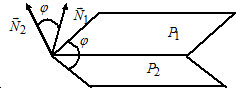
Angle between planes:

Let $P_1:A_{1}x+B_{1}y+C_{1}z+D_1=0,$ ${\bar{N}}_1=(A_1,B_1,C_1);$

$P_2:A_{2}x+B_{2}y+C_{2}z+D_2=0,$ ${\bar{N}}_2=(A_2,B_2,C_2).$

$\cos \widehat{(P_1,P_2)}=$ $\frac{{\bar{N}}_1\cdot {\bar{N}}_2}{|{\bar{N}}_1||{\bar{N}}_2|}=$ $\frac{A_1\cdot A_2+B_1\cdot B_2+C_1\cdot C_2}{\sqrt{A_1^2+B_1^2+C_1^2}\cdot \sqrt{A_2^2+B_2^2+C_2^2}}.$

Examples.

In the tasks, investigate the mutual arrangement of the given planes. In the case of $P_1\parallel P_2$, find the distance between the planes, and in the case of perpendicularity, find the cosine of the angle between them.

2.185. $P_1:-x+2y-z+1=0;$ $P_2:y+3z-1=0.$

Solution.

Let's calculate the angle between the given planes.

$P_1:-x+2y-z+1=0,\Rightarrow {\bar{N}}_1=(-1,2,-1);$

$P_2:y+3z-1=0,\Rightarrow {\bar{N}}_2=(0,1,3).$

Then

$\cos \widehat{(P_1,P_2)}=$ $\frac{{\bar{N}}_1\cdot {\bar{N}}_2}{|{\bar{N}}_1||{\bar{N}}_2|}=$ $\frac{-1\cdot 0+2\cdot 1-1\cdot 3}{\sqrt{(-1{)}^2+2^2+(-1{)}^2}\cdot \sqrt{0^2+1^2+3^2}}=\frac{-1}{\sqrt{60}}=\frac{-1}{2\sqrt{15}}.$

Thus, the planes intersect, and the cosine of the shortest angle between the planes is

$\cos \widehat{(P_1,P_2)}=-\frac{1}{2\sqrt{15}}.$

Answer: The planes intersect. $\cos \widehat{(P_1,P_2)}=-\frac{1}{2\sqrt{15}}.$

2.187. $P_1:x-y+1=0;$ $P_2:y-z+1=0.$

Solution.

Let's calculate the angle between the given planes.

$P_1:x-y+1=0,\Rightarrow {\bar{N}}_1=(1,-1,0);$

$P_2:y-z+1=0,\Rightarrow {\bar{N}}_2=(0,1,-1).$

Then

$\cos \widehat{(P_1,P_2)}=$ $\frac{{\bar{N}}_1\cdot {\bar{N}}_2}{|{\bar{N}}_1||{\bar{N}}_2|}=$ $\frac{1\cdot 0+(-1)\cdot 1+0\cdot (-1)}{\sqrt{(1{)}^2+(-1{)}^2+0^2}\cdot \sqrt{0^2+1^2+(-1{)}^2}}=\frac{-1}{\sqrt{4}}=\frac{-1}{2}.$

Thus, the planes intersect, and the cosine of the shortest angle between the planes is

$\cos \widehat{(P_1,P_2)}=\frac{1}{2}.$

Answer: The planes intersect. $\cos \widehat{(P_1,P_2)}=\frac{1}{2}.$

2.196. Construct the equation of the plane $P,$ passing through the point $A(1,1,-1)$ and perpendicular to the planes $P_1:2x-y+5z+3=0$ and $P_2:x+3y-z-7=0.$

Solution.

For the plane $P$ to be perpendicular to the planes $P_1$ and $P_2,$ it needs to be parallel to their normal vectors $N_1$ and $N_2.$ Or, equivalently, perpendicular to the cross product $[N_1,N_2]$

$P_1:2x-y+5z+3=0,\Rightarrow {\bar{N}}_1=(2,-1,5);$

$P_2:x+3y-z-7=0,\Rightarrow {\bar{N}}_2=(1,3,-1).$

$[N_1,N_2]=\left|\begin{array}{ccc}i& j& k\\ 2& -1& 5\\ 1& 3& -1\end{array}\right|=i(1-15)-j(-2-5)+k(6+1)=$ $=-14i+7j+7k.$

Now let's write the equation of the plane passing through the given point $A(1,1,-1)$ and perpendicular to the vector $[N_1,N_2]=(-14,7,7):$

$-14(x-1)+7(y-1)+7(z+1)=0÷7$

$-2(x-1)+y-1+z+1=0$

$-2x+y+z+2=0.$

Answer: $-2x+y+z+2=0.$

Homework.

In the problems, investigate the mutual arrangement of the given planes. In the case of $P_1\parallel P_2$, find the distance between the planes, and in the case they are not parallel, find the cosine of the angle between them.

2.186. $P_1:2x-y+z-1=0;$ $P_2:-4x+2y-2z-1=0.$

2.188. $P_1:2x-y-z+1=0;$ $P_2:-4x+2y+2z-2=0.$

Tags: Mutual arrangement of planes, angle between planes, geometry, plane in space