Calculation of areas and volumes using double integrals

The area of the region.

The area of the region $S$, located in the $xy$-plane, is calculated using the formula$$S=\underset{S}{\iint }dxdy.$$

Examples.

Find the area bounded by the following curves: $xy=a^2,,,x+y=\frac{5}{2}a,,,,,(a>0).$

Solution.

We find the area of the region using the formula $S=\underset{S}{\iint }dxdy.$ To compute the double integral, we first find the points of intersection of the two given curves.

$$\{\begin{array}{l}xy=a^2\\ x+y=\frac{5}{2}a\end{array}\Rightarrow \{\begin{array}{l}x=\frac{a^2}{y}\\ \frac{a^2}{y}+y=\frac{5}{2}a\end{array}\Rightarrow \{\begin{array}{l}x=\frac{a^2}{y}\\ a^2+y^2=\frac{5}{2}ay\end{array}$$

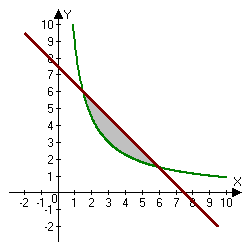
We solve the quadratic equation.

$$y^2-\frac{5}{2}ay+a^2=0$$

$$D=\frac{25}{4}{a}^2-4a^2=\frac{9}{4}{a}^2$$

$$y_1=\frac{\frac{5}{2}a+\frac{3}{2}a}{2}=2a,$$

$$y_2=\frac{\frac{5}{2}a-\frac{3}{2}a}{2}=\frac{a}{2}.$$

Accordingly, $$x_1=\frac{a^2}{2a}=\frac{a}{2},$$

$$x_2=\frac{a^2}{\frac{a}{2}}=2a.$$

Now we find the area of the figure:

$$S=\underset{S}{\iint }dxdy=\underset{a/2}{\overset{2a}{\int }}\underset{a^2/x}{\overset{\frac{5}{2}a-x}{\int }}dxdy=\underset{a/2}{\overset{2a}{\int }}(\frac{5}{2}a-x-\frac{a^2}{x})dx={(\frac{5}{2}ax-\frac{x^2}{2}-a^2\ln x)|}_{a/2}^{2a}=$$ $$=\frac{5}{2}2a^2-\frac{4a^2}{2}-a^2\ln (2a)-\frac{5}{2}\frac{a^2}{2}+\frac{a^2}{8}+a^2\ln \frac{a}{2}=5a^2-2a^2-a^2\ln (2a)-\frac{5a^2}{4}+\frac{a^2}{8}+a^2\ln \frac{a}{2}=$$ $$=\frac{15}{8}{a}^2-a^2(\ln 2+\ln a-\ln a+\ln 2)=\frac{15}{8}{a}^2-2a^2\ln 2.$$

Answer: $\frac{15}{8}{a}^2-2a^2\ln 2.$

Example.

Compute the area of the region bounded by the parabolas:

$$y^2=x,y^2=3x,x^2=y,x^2=4y.$$

Solution.

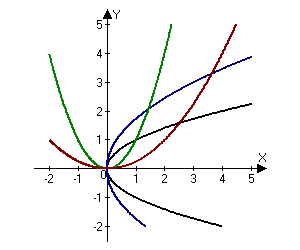
Let's introduce new coordinates $(u,v):$

$$y^2=ux,x^2=vy,$$

$$u=\frac{y^2}{x},v=\frac{x^2}{y},$$

So

$$S=\underset{D}{\iint }dxdy=\underset{G}{\iint }\frac{1}{3}dudv=\frac{1}{3}\underset{1}{\overset{3}{\int }}du\underset{1}{\overset{4}{\int }}dv=2.$$

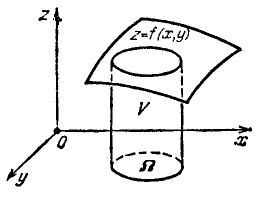
Volume of a cylindroid.

The volume of a cylindroid, bounded above by a continuous surface $z=f(x,y)\ge 0$, below by the plane $z=0$, and laterally by the straight cylindrical surface, cutting out from the $xy$-plane a measurable region $\mathrm{\Omega }$, is given by$$V=\underset{\mathrm{\Omega }}{\iint }f(x,y)dxdy.$$

Tags: Calculation of area, Mathematical Analysis, double integrals, integrals